
Statement of Inquiry
Geometry and trigonometry allow us to quantify the physical world, enhancing our spatial awareness in two and three dimensions. This branch provides us with the tools for analysis, measurement, and transformation of quantities, movements, relationships.
Concepts
Space,
Representation
Space:
The frame of geometrical dimensions describing an entity.
READ MORE >
Representation:
The manner in which something is presented.
READ MORE >
Learning Topics
-
Area of a triangle and other 2D figures
-
Area of a sector
-
3D Solids: right cylinder, pyramid,
right cone, sphere, hemisphere -
An angle between two lines and
between a line and a plane -
Volume
-
Surface area of 3D figures
Course Syllabus Topics
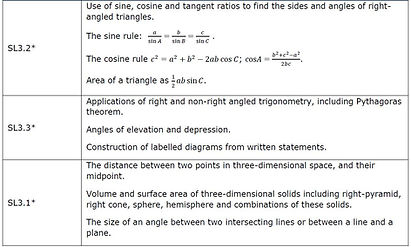
Prior Learning Support
Conceptual Understandings
-
The properties of shapes are highly dependent on the dimension they occupy in space.
-
Volume and surface area of shapes are determined by formulas, or general mathematical relationships or rules expressed using symbols or variables.
-
The relationship between the length of the sides and the size of the angles in a triangle can be used to solve many problems involving position, distance, angles , and area.
Further Conceptual Understandings

Lesson 1:
The Sine Rule
Link to the Course Calendar

In SeeSaw, respond to one. Then, comment to someone else with agreements/disagreements.
TOK Reflections:
.If sine, cosine and tangent are ratios of angles to sides in right triangles how is it possible that we use them in formulas for non-right triangles?
Reflect
Lesson 2:
The Cosine Rule

In SeeSaw, respond to one. Then, comment to someone else with agreements/disagreements.
TOK Reflections:
It is often said that Euler's relation is the most
beautiful equation in all of mathematics. What is meant by beauty and elegance in math ?
Reflect
Lesson 3:
Area of a Triangle

In SeeSaw, respond to one. Then, comment to someone else with agreements/disagreements.
TOK Reflections:
"Mathematicians admire elegance and simplicity above all else -- the ultimate goal is always the method that does the manner most efficiently." Keith Devlin Discuss.
Reflect
Lesson 4:
Sector Area

In SeeSaw, respond to one. Then, comment to someone else with agreements/disagreements.
TOK Reflections:
None today.
Reflect
Lesson 5:
Volume

In SeeSaw, respond to one. Then, comment to someone else with agreements/disagreements.
TOK Reflections:
What are the Platonic Solids and why are
they an important part of the language of
mathematics ?
Reflect
Lesson 6:
Surface Area

In SeeSaw, respond to one. Then, comment to someone else with agreements/disagreements.
TOK Reflections:
...
Reflect
Lesson 7:
Toolkit Activity
and Unit Review

Catch up on recent
videos / practice
assignments.
Ask your questions
in our Unit Discussion.
Learn

In SeeSaw, respond to one. Then, comment to someone else with agreements/disagreements.
TOK Reflections:
...


