
Units 3+4 - Linear, Quadratic, & Rational Functions

Statement of Inquiry
Models are depictions of real-life events using expressions, equations or graphs while a function is defined as a relation or expression involving one or more variables. Creating different representations of functions to model the relationships between variables, visually and symbolically as graphs, equations and tables represents different ways to communicate mathematical ideas.
Concepts
Modelling,
Relationships, Representation,
Equivalence
Models: Depictions of real-life events using expressions, equations, and graphs.
READ MORE >
Relationships: the connections and associations between properties, objects, people, and ideas -- including the human community's connections with the world in which we live. READ MORE >
Representation: The manner in which something is presented. READ MORE >
Equivalence: The state of being identically equal to or interchangeable, applied to statements, quantities, or expressions. READ MORE >
Learning Topics
-
Domain and Range of a Function
-
Inverse and Composite Functions
-
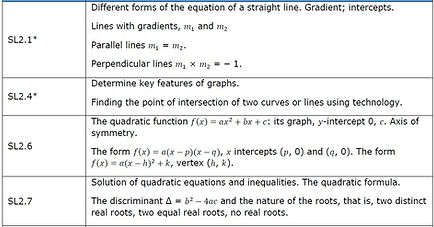
Features of a Parabola: symmetry, vertex,
intercepts, equation of the axis of symmetry -
Forms of a Quadratic Function:
standard (general), intercept, vertex -
Factorization and completing the square
-
Roots of an equation/zeros of a function
-
Discriminant
-
Transformations of functions:
reflections, stretches, and translations -
Features of reciprocal and rational functions: symmetry, intercepts, horizontal and vertical asymptotes
-
modelling with reciprocal and rational functions
Course Syllabus Topics


Prior Learning Support
Prior Learning 1 - Solving Linear Equations
Prior Learning 2 - Expanding Brackets and Factoring
Prior Learning 3 - Factorizing Quadratic Expressions
Prior Learning 4 - Factorizing a Difference of Squares

Conceptual Understandings
-
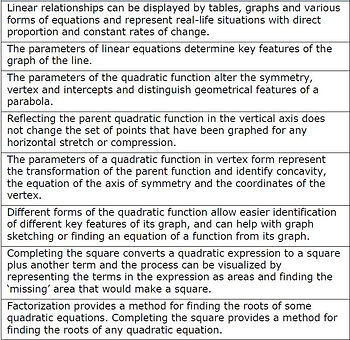
Different representations of functions, symbolically and visually as graphs, equations and tables provide different ways to communicate mathematical relationships.
-
The parameters in a function or equation correspond to geometrical features of a graph and can represent physical quantities in spatial dimensions.
-
Moving between different forms to represent functions allows for deeper understanding and provides different approaches to problem solving
-
Equivalent representations of quadratic functions can reveal different characteristics of the same relationship.
Further Conceptual Understandings
Unit Discussion
Questions & Suggestions
Click on the microphone icon to enter our
Unit and Lesson Discussion.
-
Begin a discussion thread by adding a specific
question under a Lesson heading. -
Suggest links to videos and websites that have
helped you in your learning.

Link to the Course Calendar
Lesson 1:
Parameters + Forms
of Linear Equations

In SeeSaw, respond to one. Then, comment to someone else with agreements/disagreements.
TOK Reflections:
Descartes showed that geometric problems could be solved algebraically. What does this tell us about mathematical representation and knowledge?
Reflect
Lesson 2:
Transformations
of Functions
Lesson 3:
Quadratic
Functions

Video Lesson
+ Video Notes Page
Did you finish the
Class Problem Set
for Lesson 1?
Ask your questions
in our Unit Discussion.
Learn

In SeeSaw, respond to one. Then, comment to someone else with agreements/disagreements.
TOK Reflections:
None today.
Reflect

In SeeSaw, respond to one. Then, comment to someone else with agreements/disagreements.
TOK Reflections:
How would you choose which form to use?
When is intuition helpful or hurtful in math?
Reflect
Lesson 4:
Creating
Quadratic Models

In SeeSaw, respond to one. Then, comment to someone else with agreements/disagreements.
TOK Reflections:
How can you deal with the ethical dilemma
of using mathematics to plot the course
of a missile or a bomb?
Reflect
Lesson 5:
Completing
the Square

In SeeSaw, respond to one. Then, comment to someone else with agreements/disagreements.
TOK Reflections:
None today.
Reflect
Lesson 6:
Quadratic Formula
& the Discriminant

In SeeSaw, respond to one. Then, comment to someone else with agreements/disagreements.
TOK Reflections:
None today.
Reflect
Lesson 7:
Quadratic Inequalities
& Applied Problems

In SeeSaw, respond to one. Then, comment to someone else with agreements/disagreements.
TOK Reflections:
None today.
Reflect
Lesson 8:
Reciprocal Functions
and Transformations

Video Lesson 1
+ Notes Page
Video Lesson 2
+ Notes Page
Ask your questions
in our Unit Discussion.
Learn

In SeeSaw, respond to one. Then, comment to someone else with agreements/disagreements.
TOK Reflections:
None today.
Reflect
Lesson 9:
More Transformations
of Rational Functions

In SeeSaw, respond to one. Then, comment to someone else with agreements/disagreements.
TOK Reflections:
None today.
Reflect
Lesson 10:
Unit Review

See the many review
activities in the
"Collaborate" section.
Ask your questions
in our Unit Discussion.
Learn

Start here:
Video Lesson:
Re-Teaching using the Formative Assessment
Need More Practice?
Try another practice assessment with video tutorials to guide you...
Practice Assessment 1
--> Support Video 1
--> Support Video 2
--> Support Video 3
Practice Assessment 2
+ Answer Key
Review Assignments:
--> Linear/Quadratic
--> Rational Functions
Collaborate

In SeeSaw, respond to one. Then, comment to someone else with agreements/disagreements.
TOK Reflections:
None today.
